x
x px >
px >  , where
, where  denotes the uncertainty, x is the position of the point mass m along the x-axis,
denotes the uncertainty, x is the position of the point mass m along the x-axis, px = m vx is the momentum along the x-axis, vx is the velocity along the x-axis, and
 = h/2
= h/2 = 1.054x10-27 erg-sec. A similar relation exists for the uncertainty of the time t and energy E, e.g.,
= 1.054x10-27 erg-sec. A similar relation exists for the uncertainty of the time t and energy E, e.g., Figure 12-02 Uncertainty Principle [view large image]
 t
t  E
E 
 . In case of heavy mass (such as a macroscopic object), the uncertainties and thus the quantum effect becomes very small, classical physics is applicable once more.
. In case of heavy mass (such as a macroscopic object), the uncertainties and thus the quantum effect becomes very small, classical physics is applicable once more. See "A Derivation of the Uncertainty Principle" for more detail.

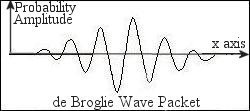
 = h/p, which is known as the de Broglie wavelength. Since there is a spread of momentum according to the uncertainty principle, the electron wave is described by a wave
= h/p, which is known as the de Broglie wavelength. Since there is a spread of momentum according to the uncertainty principle, the electron wave is described by a wave