Home Page Overview Site Map Index Appendix Illustration About Contact Update FAQ


Molecules

Covalent Bond, Hydrogen Molecule

H2 Wave Function Potential Curve When two hydrogen atoms approach each other, the final configuration depends on the spin of the two electrons (a consequence of the Exclusion Principle). If the spin of the two electrons is parallel as shown in the right side of Figure 12-08, the two atoms remain separated. However, if the spin of the two electrons is antiparallel as shown in the left side of Figure 12-08, the two atoms combine to form a hydrogen molecule. There is a high probability of finding the electrons in between the atomic nuclei and this "electron cloud" tends to keep them from breaking up. This kind of binding is called covalent bond, and is purely

Figure 12-08 H2 Wave Function
[view large image]

Figure 12-09 H2 Potential Curve [view large image]

 a quantum effect. Figure 12-09 shows the electronic energy as a function of inter-nuclear separation. It is usually referred to as potential curve, which can be
derived either theoretically from the numerical solution of the Schrodinger equation or experimentally by analyzing the spectroscopic data. The potential curve UA is repulsive while US forms a potential well to keep the atomic nuclei within unless they absorb a quantum of light with energy more than the dissociation energy of 4.52 ev.

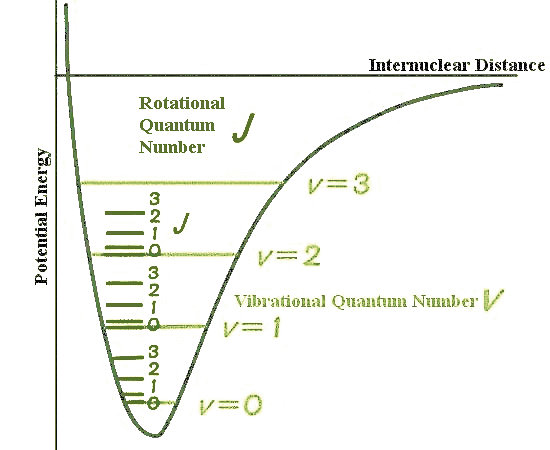
In presenting the potential curve for a diatomic molecule, one of the atomic nucleus is usually fixed at the origin of the coordinate frame. The other nucleus is then portrayed as vibrating and rotating inside the potential well as shown in Figure 12-10a. The vibration is restricted to discrete energy levels. Each of the vibrational energy level v is further split into a series of rotational energy levels J called vibrational band. Wave functions are shown in two vibrational energy levels in Figure 12-10b. According to classical physics, particle cannot penetrate the potential wall; however, in quantum there is a certain probability of infiltration (tunneling) outside the wall (see
Energy Levels Transition Figure 12-10b). In general, transitions are favored by superimposing an initial configuration of high probability with a final one of high probability as shown by the green line in Figure 12-10b. Transitions are also governed by selection rules, which usually allow transitions to occur only between change of the rotational or vibrational quantum number by an amount that amount - one step at a time. 1. The former is related to the initial and final states of the molecule, which favors the one step change in the rotational configuration. While the latter is linked to the oscillating state of the molecule, which occurs only at certain resonant energy so that the emitting or absorbing photon can carry only that amount - one step at a time.

Figure 12-10a Vibrational and Rotational Energy Levels [view large image]

Figure 12-10b Transition [view large image]

 See "Franck-Condon" principle for electronic-vibrational transition.

Go to Next Section
 or to Top of Page to Select
 or to Main Menu

.