Quantum Field Theory

Spontaneous Symmetry Breaking

The existence of asymmetric solutions to a symmetric theory is common to many branches of physics. The reason lies in the fact that the symmetric state is not the state of minimum energy, i.e., the ground state, and that in the process of evolving towards the ground state, the intrinsic symmetry of the system has been broken. Figure 06e shows that the initial position of the marble on top of the bump is symmetric but not in a state of minimum energy. A small perturbation will cause the rotational symmetry to be broken and the

Figure 06e Symmetry Breaking

system to assume a stable state configuration. When the symmetry of a physical system is broken in this way, it is often referred to as "spontaneous symmetry breaking".

This idea can be applied to the theory of cosmic inflation or to account for the mass of elementary particles in the Standard model via interaction with the Higgs boson as shown below. Let us start by examine how such unstable symmetry can arise mathematically. Considering the Lagrangian for a scalar field with the potential V:

L = (1/2) - V() ---------- (46)

where V() = (1/2)m²² + (

/4!)⁴, and 4! = 4x3x2x1.

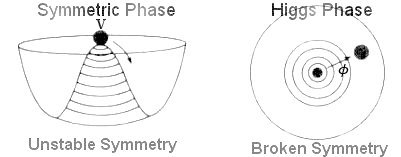
Figure 06f Scalar Field
[view large image]

If m² > 0, the system has real mass, the potential exhibits a minimum at the origin, where = 0. This system is associated with a unique vacuum (see Figure 06f).

When m² < 0, the vacuum at = 0 is unstable; a particle would prefer to move down the potential to a lower-energy state at the bottom of one of the wells at = v = (-6m²/

)^1/2. The states at = 0, and = v are referred to as false vacuum, and true vacuum respectively (Fiigure 06f). Eq.(46) indicates that the system is symmetrical under

-. If the origin at the false vacuum is shifted to v, i.e., ' = - v, then the Lagrangian in Eq.(46) becomes:

L = (1/2)'' - V'(') ---------- (47)

with V'(') = |m|²'² + (1/6)

v'³ + (

/4!)'⁴. In this way, the scalar particle has acquired a positive mass squared given by 2|m|², but the original symmetry between and - has been spontaneously broken because the field has been shifted (resulting in the occurrence of the '³ term) and there is a true vacuum at ' = 0. Note that the valley in diagram (b) has a depth of (3/2)(m⁴/

). The zero point of the potential can be shifted up or down without any effect on subsequent calculation.

	The formulation can be generalized to complex scalar field with two independent components corresponding to positively and negatively charged fields. In a slightly different notations, the Lagrangian for a complex scalar field has the form similar to Eq.(46): L = ()() - V(,) ---------- (48) where V() = m²* + (/4)(*)².
Figure 06g Complex Scalar Field [view large image]

In this case there is a circle of degenerate minima giving by (Re)² + (Im)² = v², where v = (-2m²/

)^1/2 (see Figure 06g). Therefore, there are infinitely many possibilities for the stable configuration: any scalar field satisfying the condition for minimum energy will do. The Lagrangian in Eq.(48) is invariant under the global gauge transformation:

= (₁ + i₂)eⁱ/(2)^1/2

which is just a rotation in the ₁-₂ plane. In analogy to the previous example, the origin of one of the field components, e.g., ₁ is shifted to a point at the circle of minimum energy:
= ₁ - v
= ₂
In terms of , and , the Lagrangian is transformed to:

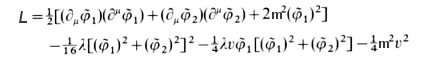
Once again, the symmetry is spontaneously broken as before. The mass term can be easily located by looking for the one with quadratic fields. It reveals that the field acquires a (positive) mass squared of -2m². The novel feature in this example is that the field remains massless. Such massless modes, which arise from the degeneracy of the ground state after spontaneous symmetry breaking, are called "Goldstone bosons". The appearance of Goldstone bosons seems to be in contrary to real world experience since no such massless, spin-0 particle exists.

However, if the global gauge transformation in the formulation is replaced by local gauge transformation, it can be shown that the Goldstone boson is absorbed by the formerly massless gauge boson, which has now acquired a mass. The corresponding model is called "scalar electrodynamics", but when it it spontaneously broken it is then referred to as the "Higgs mechanism". Before the symmetry breaking the Lagrangian for the interacting scalar field and electromagnetic vector potential A has the form:

If the vector potential is transformed as:

where q is the coupling constant and v is the scalar field at the true vacuum as defined earlier. By a suitable choice of local gauge transformation such as:

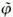' = e^-iq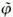, with q

= tan^-1(/)

then the scalar field components become:

' = H = cos(q

) + i sin(q

)
' = 0

The Lagrangian re-emerges in the form:

which shows that the symmetry is broken (by the odd power terms in H), and the Goldstone mode has been completely removed by the gauge-transformed boson A', which has acquired a mass qv. The remaining scalar field H has also acquired a mass (-2m²)^1/2. The total number (four) of degrees of freedom is unaltered. Instead of a massless gauge boson, having two (transverse) modes, plus a complex field composed of two real components, we now have a massive vector field A' having three modes - two transverse and one longitudinal (a requirement for massive spin-1 boson), plus one real scalar field H. This is just a theoretical model to illustrate the effect of spontaneous symmetry breaking. Of course, the photon remains massless in the real world. It requires both spontaneous symmetry breaking (with v

0) and the coupling of the gauge field to the scalar field (q

0) to acquire a mass. A more complicated version of this model is applicable to the electroweak interaction in the Standard model. It has been shown subsequently by 't Hooft that the spontaneous symmetry breaking formulation remains renormalizable; the ultraviolet divergences encountered are no worse than those occurring in QED.

In the Standard model the scalar field is identified as the Higgs field responsiable for the mass of fermions and gauge bosons. Supersymmetry increases the number of Higgs particles to five with masses ranging from about 100 - 400 Gev. The Higgs fields are supposed to permeated throughout the universe uniformly and isotropically since the Big Bang. The spontaneous symmetry breaking occurred at a temperature corresponding to about 250 Gev in the electroweak ear soon after the end of inflation. Above that temperature (the phase is known as the symmetric phase) all the particles become massless. The period after the transition is called the Higgs phase. In a way, the Higgs fields are similar to the hypothetical ether of the pre-relativity era, but with a crucial difference - that it is formulated as a relativistic invariant theory, which would not prescribe an absolute frame of reference.

As each term in the Lagrangian of the Standard model represents a different process, Figure 06h shows the various Higgs interactions in the form of Feynman diagrams. Diagram (a) represents a fermion emitting or absorbing a Higgs particle. Diagram (b) shows the corresponding process for the gauge bosons. They can also interact simultaneously with two Higgs, as shown in (c), which also represents a gauge boson scattering a Higgs particle. The Higgs also interacts with itself, as shown in diagrams (d) and (e), which are related to the shape of the scalar potential (Figure 06g). Diagram (f) depicts an electron acquiring its mass.

Figure 06h Higgs Field Interaction

	By taking into account the higher term of the SM (Standard Model) perturbation series, the vacuum potential of the Higgs field V is in the form :
Figure 06i Stability of the Universe [view large image]	and the sum is over all SM particles acquiring a Higgs-dependent mass M_i. The precise form of V₁ is not important in the present context, it just shows that the Higgs potential also depends on the particles it acts upon. Furthermore, only the heaviest top quark in the sum is retained in the following consideration.

As shown in Figure 06i,a and the above formula, the vacuum potential V depends on the mass of the Higgs m and also the mass of the top quark m_t. Computation of V with the various values of m and m_t yields different forms, which can be stable, meta-stable, or runaway (see Figure 06i,a). Figure 06i,b plots the result revealing our universe is possibly in an meta-stable state sitting on the verge of catastrophic decay into the true vacuum. The revelation produced sensational news headline around the globe one morning in 2013 (soon after the discovery of Higgs in July, 2012). A second wave of doomsday prophecy occurred in 2014 with Hawking's blessing on the revelation.