

| Home Page | Overview | Site Map | Index | Appendix | Illustration | About | Contact | Update | FAQ |
 |
 |
Most models of early universe assume that time starts with the Big Bang. Study of a recent theory reveals that it may not be so. Time emerged gradually along with a sequence of events as illustrated in the followings. The novel features include a "rediscovery" of the Wheeler–DeWitt equation which suggests the disappearance of time in the formulation some 50 years ago. A simplified derivation for quantization of the Friedmann Equation (Matter-only) shows the same conclusion about timeless but also reveals a mechanism that can mimic the |
Figure 01 Cosmic History and Planck Scale [view large image] |
Figure 02 Interactions, 4-types |
phenomenon of inflation. Here's the mathematical formalism for such interpretation. |
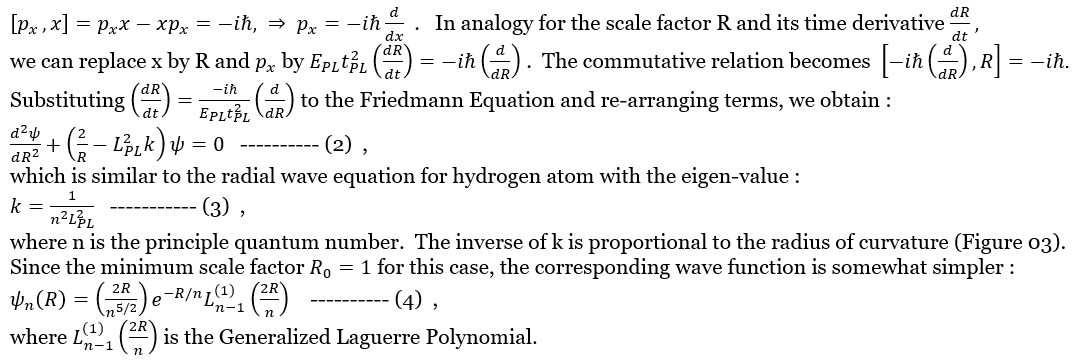
 PL ~ 10114 erg/cm3. Such entity is at the realm of quantum gravity. Unfortunately, there is no such universally accepted theory for now. One of the ad hoc solutions is to quantize the Friedmann Equation for cosmic expansion.
PL ~ 10114 erg/cm3. Such entity is at the realm of quantum gravity. Unfortunately, there is no such universally accepted theory for now. One of the ad hoc solutions is to quantize the Friedmann Equation for cosmic expansion.  (r0)3] = -kc2 ---------- (1a),
(r0)3] = -kc2 ---------- (1a), (tPL)2] = -kc2 ---------- (1b).
(tPL)2] = -kc2 ---------- (1b).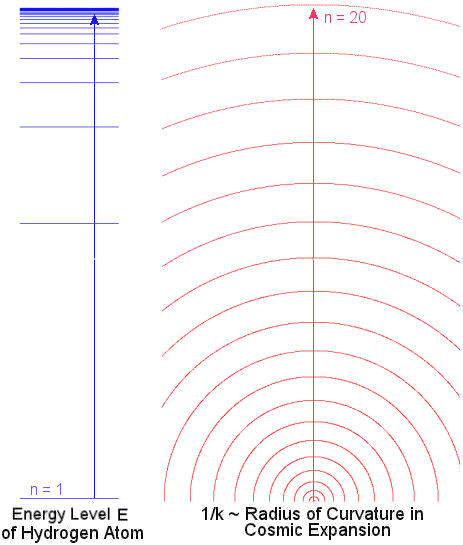 |
|
Figure 03 H Atom Energy Level & Cosmic Curvature |
See more cumbersome detail in the original derivation of "Quantization of the Friedmann Equation (Matter-only)" in 2011. |
 and k
and k  0, that's when time began and standard (classical) cosmology took over.
0, that's when time began and standard (classical) cosmology took over. |
 |
up and vanishing briefly and incessantly according to the Uncertainty Principle  t t E E   (Figure 05). The (Figure 05). The  t is an arbitrary time interval. There is lot of confusion about the meaning of time. Especially, about the time in quantum theory, which defines time as a variable different from space; while in general relativity, time is at the same level as space and is malleable (see "Quantum Time"). t is an arbitrary time interval. There is lot of confusion about the meaning of time. Especially, about the time in quantum theory, which defines time as a variable different from space; while in general relativity, time is at the same level as space and is malleable (see "Quantum Time").
|
Figure 04 Quantum Fields |
Figure 05 Virtual Particles [view large image] |
Anyway, Quantum fluctuation has profound effect on the large structure such as super galactic cluster in the later epoch of the universe. |
 PL = EPL/[(4
PL = EPL/[(4 /3)(LPL)3] = 10114 erg/cm3 persists during the quantum expansion. The mechanism could be similar to the virtual particle contribution to constant vacuum energy density, which seems to be a viable explanation in the very early universe (see Figure 06). However it is too large for standard cosmology. The problem could be with applying a quantum concept to classical physics in identifying this
/3)(LPL)3] = 10114 erg/cm3 persists during the quantum expansion. The mechanism could be similar to the virtual particle contribution to constant vacuum energy density, which seems to be a viable explanation in the very early universe (see Figure 06). However it is too large for standard cosmology. The problem could be with applying a quantum concept to classical physics in identifying this  PL to dark energy. The ad hoc merging does not always work.
PL to dark energy. The ad hoc merging does not always work. (RSh)2/(LPl)2],
(RSh)2/(LPl)2], (kBln2) ~ 4
(kBln2) ~ 4 kB. This is the lowest amount of entropy at the beginning of the universe. It resolves the astronomical problem about the origin of low cosmic entropy. BTW, the cosmic entropy for today is about 10103 kB according to Ethan Siegel. This amount can be estimated from the average galactic RSh of about 1013 cm and the number of galaxies in present day of ~ 2x1012. About S ~ 1096 kB (for
kB. This is the lowest amount of entropy at the beginning of the universe. It resolves the astronomical problem about the origin of low cosmic entropy. BTW, the cosmic entropy for today is about 10103 kB according to Ethan Siegel. This amount can be estimated from the average galactic RSh of about 1013 cm and the number of galaxies in present day of ~ 2x1012. About S ~ 1096 kB (for  ~ 10112 erg/cm3, T ~ 5x1030 K, and V ~ 1 cm3) was infused during the "inflation" as shown in Figure 06. It has been increased ever since as the universe expands.
~ 10112 erg/cm3, T ~ 5x1030 K, and V ~ 1 cm3) was infused during the "inflation" as shown in Figure 06. It has been increased ever since as the universe expands. kB,
kB, kB = 5x1030 K = 4x1017 Gev (see "A History of Cosmic Expansion" for comparison).
kB = 5x1030 K = 4x1017 Gev (see "A History of Cosmic Expansion" for comparison). marks the transition from quantum to classical cosmological model, which involves time (see Eq. (1)). This boundary is the "new" absolute zero time (Figure 01). It shifts the conventional cosmic time scale up by an negligible amount ~ 10-33 sec. This instance could be associated with the appearance of particles, the emergence of strong interaction, and chiral symmetry breaking (Figure 02, also see "Naturalness, Chiral Symmetry, and Spontaneous Chiral Symmetry Breaking").
marks the transition from quantum to classical cosmological model, which involves time (see Eq. (1)). This boundary is the "new" absolute zero time (Figure 01). It shifts the conventional cosmic time scale up by an negligible amount ~ 10-33 sec. This instance could be associated with the appearance of particles, the emergence of strong interaction, and chiral symmetry breaking (Figure 02, also see "Naturalness, Chiral Symmetry, and Spontaneous Chiral Symmetry Breaking").  |
 |
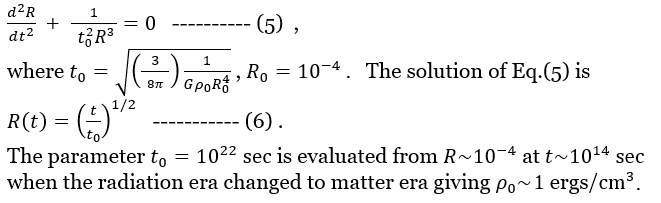
|
Figure 06 Energy Density Evolution [view large image] |
Figure 07 Cosmic History, Very Early |
Figure 06 shows the evaluation of the t0 and  0 parameters at the recombination point, while Figure 07 is a rough sketch for the evolution of the scale factor in very early universe. 0 parameters at the recombination point, while Figure 07 is a rough sketch for the evolution of the scale factor in very early universe. |
 |
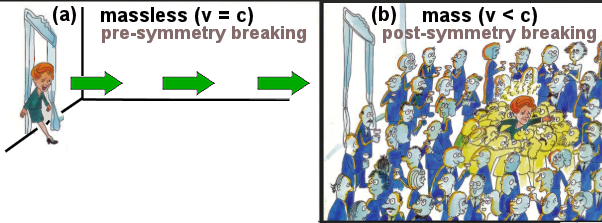 |
The same sketch also shows a very brief period of the order 10-10 sec when all the particles are massless. The Standard Model (SM) of elementary particles dictates that before the so-called "Electro-weak Symmetry Breaking", the Higgs field existed in false vacuum and could not interact with other particles making them massless (Figure 08,a) and all of them move at the speed of light (Figure 09,a). |
Figure 08 SM, Parameters |
Figure 09 Mass, Origin of |
See "Largranian for WS Model of SM", WS stands for Weinberg-Salam - two of the 1979 Nobel prize recipients for formulating the Stand Model. The third one is SG, who produced the QCD. |
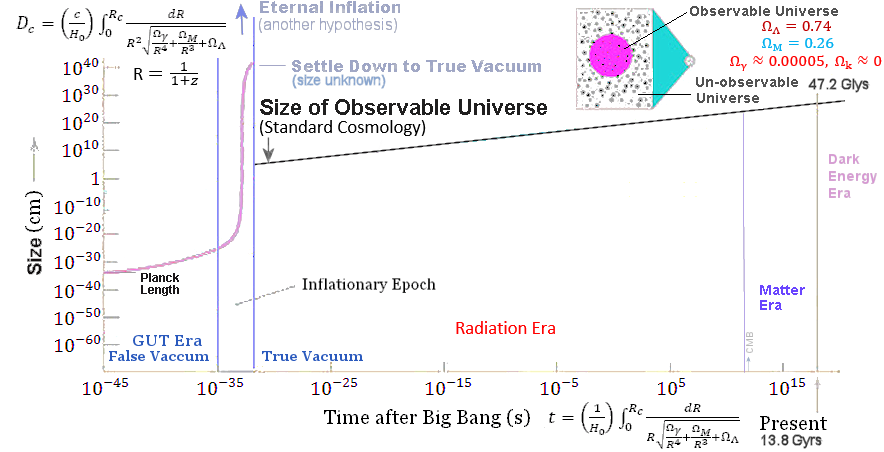 |
BTW, As shown in Figure 08, this is also the point when the electro-weak interaction separated into the weak (with massive mediating bosons - making the force short range) and electromagnetic (with the massless photon - producing long range force) interactions. The size of the universe at t ~ 10-32 sec is R(t)x(size at current epoch) ~ 10-27x1028 cm = 10 cm. This is actually the size of observable horizon such as the one shown in Figure 07. The inflation could go beyond to the un-observable part as illustrated in Figure 10. |
Figure 10 Cosmic Expansion |
 |
In special relativity, the space-time interval ds is an invariance under the Lorentz transformation with ds2 = c2dt2 - (dx2 + dy2 + dz2). Since ds  0 for light wave as demanded by the theory, dt2 = (dx2 + dy2 + dz2)/c2 which shows that time is not an independent variable, it is proportional to the distance before the electro-weak transition. The proper time 0 for light wave as demanded by the theory, dt2 = (dx2 + dy2 + dz2)/c2 which shows that time is not an independent variable, it is proportional to the distance before the electro-weak transition. The proper time  is defined by the clock sitting at x = y = z = 0, or ds is defined by the clock sitting at x = y = z = 0, or ds  cd cd = cdt. For an external observer moving with a relative velocity v, d = cdt. For an external observer moving with a relative velocity v, d = dt[1-(v/c)2]1/2 (Figure 11). Since v = c for all particles in that period, hence cd = dt[1-(v/c)2]1/2 (Figure 11). Since v = c for all particles in that period, hence cd  ds ds  0 as defined previously. 0 as defined previously.
|
Figure 11 |
Accordingly, time can be defined as the rate of change R (for anything), e.g., on a particle's position R = dx/dt; beforce the electro-weak symmetry breaking, R = c; R  c afterward depending on the interaction between the particle and Higgs. Such R is usually referred to as velocity v, the closely related entity is the linear momentum mv. c afterward depending on the interaction between the particle and Higgs. Such R is usually referred to as velocity v, the closely related entity is the linear momentum mv. |