Bell's Theorem (Quantum vs Classical)

The 2022 Nobel prize in physics has been awarded to A. Aspect, J. Clauser and A. Zeilinger for their experiments on Bell's Theorem verifying the validity of quantum theory. It is confirmed by their works laying down the foundations for a plethora of quantum technologies, including quantum computers and communications (see "Spooky quantum-entanglement experiments win physics Nobel").

In 1935, Einstein, Podolsky, and Rosen proposed a paradox (hence EPR-paradox) as a thought experiment ("gedankenexperiment" in German) in which the particles can have both their position and momentum accurately measured in violation of the uncertainty principle

q >

; it also violates the principle of locality (signal cannot travels faster than the speed of light) in special relativity (see Figure 01). They argued that "elements of reality" such as momentum and position are deterministic properties of particles although it is hidden in quantum theory which endows them with probability only. Hence quantum theory is an incomplete theory, there is no need to invoke "non-locality" and also can do away with the "uncertainty principle" if the hidden cause is included in the theory.

Figure 01 EPR Paradox [view large image]

The thought experiment became real with the discovery of entanglement in 1967. Entanglement occurs for example, in the decay of

the pi meson into an electron-positron pair (Figure 02). Since the spin for the pi meson is 0, the spin for the electron-positron pair must be opposite according to the conservation of angular momentum. Therefore, no matter how far apart are the members of this pair, if the spin is flipped for one of the member, the spin for the other member will also be flipped to the opposite at precisely the same moment. This non-local influence (objects can influence each other only locally according to classical physics) occur instantaneously, as if some form of communication, which Einstein called a "spooky action at a distance", operates not just faster than the speed of light, but infinitely fast.

Figure 02 Entanglement [view large image]

Figure 02 demonstrates the origin of entanglement and its final collapse by measurement. The observers in the names of Alice and Bob are modern inventions.

Then a seminal paper by John Bell in 1964 shows that if EPR were correct, the results found by two widely separated detectors measuring certain properties of the two entangled photons (or particles) (such as the polarization direction or spin orientation about various randomly chosen detecting angle) would have to stay inside certain range - this is known as the Bell's theorem, or Bell's inequality. Starting in early 1970s, technology has improved significantly to enable the required experiments for resolving the paradox. It culminated in the early 1980s, when the Aspect experiment firmly established that measurements from the two detectors could be outside the range, i.e., violating the Bell's inequality. Quantum mechanics survived the test and entanglement will stay with us into the quantum computing age.

"Bell's Theorem : the Naive View of an Experimentalist"

As shown in Figure 03, a source S emits a pair of photons with different frequencies 1 and 2 along the z-axis. In entanglement, this pair is in the "Maximally Entangled Bell States" :

|(1, 2) = (|x,x + |y,y)/(2)^1/2 ----- (1),

where x, y denote the linear polarization direction of the 2 photons, the 1st letter refers to photon 1, while the 2nd to photon 2. In this case photons 1 and 2 always have the same polarization. The analyser I, in orientation a, is followed by two detectors to un-entangle the pair, giving results + or -, corresponding to a linear polarization found parallel or perpendicular to a. The analyser II, in orientation b, acts similarly.

	In quantum mechanics, the probabilities of joint detections of 1 and 2 in the channels + or - of polarisers I or II can be expressed by : P₊₊() = P_--() = cos²()/2 ----- (2a) P_+-() = P_-+() = sin²()/2 ----- (2b)
Figure 03 Bell's Inequality Test, Single Channel [view large image]	where = ab denotes the angle between the analysers I and II.

The correlations between random measurements can be expressed in term of the correlation coefficient :
E_QM() = P₊₊() + P_--() - P_+-() - P_-+() ----- (3).
Thus E_QM = cos(2) ----- (4)
by substituting Eqs.(2a,b) into Eq.(3). Eq.(4) shows that the correlation is total for = 0 as E_QM(0) =1.

	It is shown in the above-mentioned original paper that the classical (EPR) correlation coefficient has the form : E_EPR() = 1 - (4)/ ----- (5) Figure 04 compares the difference between E_QM and E_EPR. It is always small, and there are agreements at = 0, /4, /2.
Figure 04 Correlation Coefficient [view large image]

A more sensitive test for the difference involves two directions of simultaneous analysis for each analyer (a and a' for I, b and b' for II, see Figure 05). Correlation functions corresponding to these orientations ("settings") are combined to a new quantity:
S(a,a',b,b') = E(a

b) - E(a

b') + E(a'

b) + E(a'

b') ----- (6).
It can be shown (in that paper again) that for the case of hidden variable, the values of S are restricted to a range :

Figure 05 2-channel Bell Test
[view large image]

-2

S_EPR(a,a',b,b')

Bell's Inequality ----- (7)
In practice, the angles between the analyers are chosen to be 0^o, 45^o, 22.5^o, and 67.5^o.

	By substituting Eq.(4) into Eq.(6), and for a particular orientation of the analyer angles (see Figure 06), we obtain the maximum violation of : S_QM = 2(2)^1/2 = 2.8284 ----- (8) which is clearly outside the range defined by S_EPR in Eq.(7). Figure 06 shows the variation of S_QM over a range of and the regions of violation.
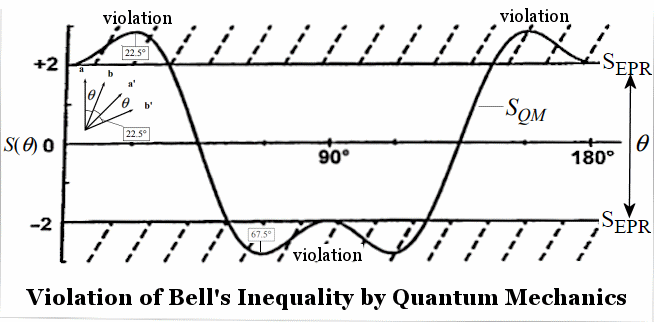
Figure 06 Bell's Inequality [view large image]	The Aspect experiment obtains S_exp = 2.697 0.015 to validate Quantum Mechanics as a correct theory.

The Bell's inequality violation mentioned so far does not involve nonlocality (the "spooky action" faster than the speed of light).

The distance between the measuring analyers has to be space-like, meaning that the signal to indicate the matching of 2 events has to travel faster than the speed of light. There is a coincidence monitor to make sure the signals arriving at almost the same time.
The choices of the events must be at random. For example, if both analyers are fixed to measure parallel polarizations, then polarization from different pair can be matched, the checking becomes meaningless.

The setup of such experiment by Aspect is illustrated in Figure 07 :

The separation of the 2 analyers (at system I and II) is 1300 cm. It needs 43 ns to travel between them at speed of light.
Variation of the analyers is simulated by optical switch C which directs the photons to 2 different analyers. The change of orientation of the equivalent variable polarizer then occurred after inequal intervals of 6.7 ns and 13.3 ns. This is one of the problem with the experiment because the switching is not truly random.
The delay between the emissions of the two photons of a pair is about 5 ns on average. Any match registered by the coincidence monitor would suggest faster than light signal with velocity ~ 2.6x10¹¹ cm/sec (~ 10 times the speed of light).

	The Bell's inequality for this case is : -1 S'_EPR 0 Bell's Inequality for Locality ----- (9) which just reiterates the fact that events would not be recorded if the signal is not faster than the speed of light.
Figure 07 Non-locality Experiment [view large image]

The experimental result is S'_exp = 0.101

0.020, comparing to the quantum mechanical prediction of S'_QM = 0.113

0.005 in support of nonlocality. It is admitted that the experiment is far from ideal on many points. Thus, it leaves open a loophole of whether violation of Bell's inequality implies nonlocality as well. Actually, the answer depends on which one of the 2 Bell's inequalities - the one in Eq.(7) only check out the EPR paradox (if QM is incomplete), while Eq.(9) is specifically for the determination of locality/non-loacality in addition to check out the proposition of hidden variable.

N.B. This kind of spooky signal does not convey information - a caution by all physicists.

	An equilvalent form of the Bell's inequality asserts that if EPR were correct, the results found by two widely separated detectors measuring certain properties of 2 entangled particles would have to agree (match) more than 50% of the time. Figure 08 provides a very specific example to illustrate how underlying pre-arrangement (hidden variables) can bump up the chance of QM ramdon match.
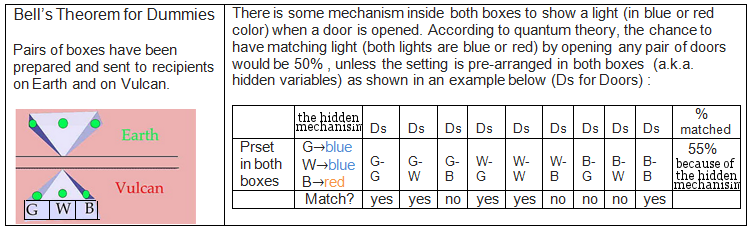
Figure 08 Bell's Theorem for Dummies [view large image]	This is Brian Greene's analogy also known as "Bell's Theorem for Dummies".

The tests on the Bell's theorem have at least two loopholes until 2015 when new technology allows experiment to skirt such problems. One problem is known as the "detection loophole" with the lost up to 80% of the entangled qbits (in the forms of photons) and it is not sure that the remaining 20% is the true representative. The use of closely spacing atoms may retain more qbits for the measurements, but it could be masked by under speed of light communication, and it thus earns the name as "communication loophole". The 2015

experiment at Delft University solves both problems as shown in Figure 09. It produces entanglement of two electrons inside two diamonds respectively (separated by 1.3 km - enough to close the communication loophole and with no lost of entangled qbits) via the entanglement of the photons emitted by each. The occurrence is not very often - just a few per hour. Eventually, 245 measurements were taken to show that the standard quantum view is valid. Difficulty of the experiment produced a p-value of only 4% - a statistical significance just passes the usual 5% and is much shorter than the 1/10⁶ standard for experiment in physics. Anyway, this experiment also guarantees the security in "quantum cryptography", which may be hacked through the loopholes.

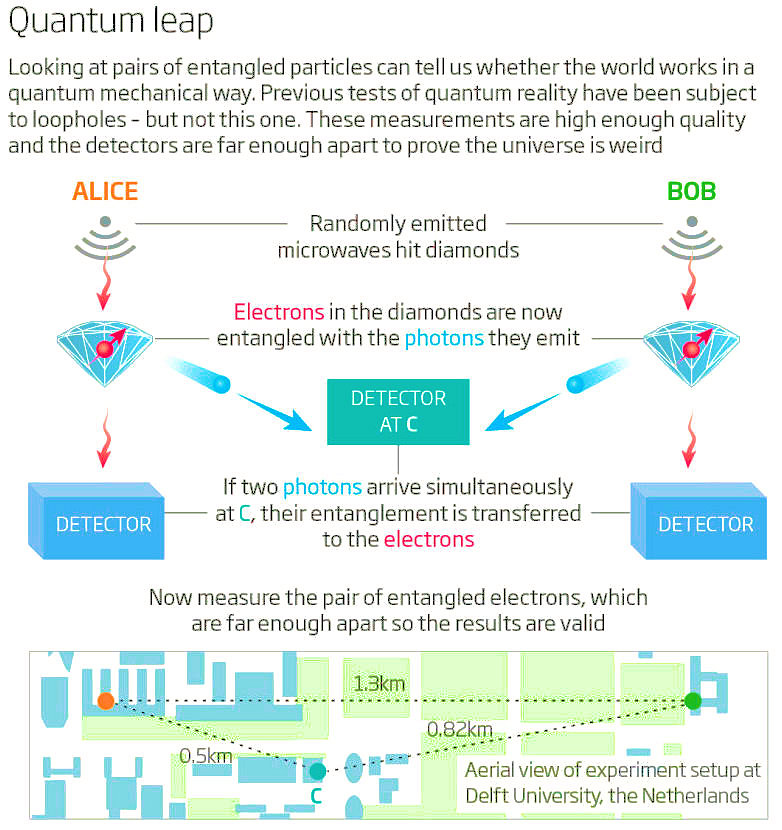
Figure 09 Bell Test, 2015

See more detail in "Quantum 'spookiness' passes toughest test yet".

One argument for "hidden variables" suggests that the hidden links were established long time ago; it is there already before the measurement, free-will or random number generator in the measuring process notwithstanding. A 2017 experiment using star light (red, or blue) to trigger the measurement shows that quantum entanglement comes with the "spooky action at a distance" property, i.e., no "hidden variables" to link the pair as far back as 600 years ago when the star light was emitted .

Finally, see "Bell Test Experiments" over the years.