Higgs Field, the Eternal and Ubiquitous (2021 Edition)

Contents

Spontaneous Symmetry Breaking (SSB) and Phase Transition

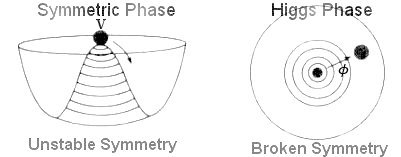
The existence of asymmetric solutions to a symmetric theory is common to many branches of physics. The reason lies in the fact that the symmetric state is not the state of minimum energy, i.e., the ground state, and that in the process of evolving towards the ground state, the intrinsic symmetry of the system has been broken. Figure 01 shows that the initial position of the marble on top of the bump is symmetric but not in a

Figure 01 Symmetry Breaking

state of minimum energy. A small perturbation will cause the rotational symmetry to be broken and the system to assume a stable state configuration. When the symmetry of a physical system is broken in this way, it is often referred to as "spontaneous symmetry breaking (SSB)".

		process of SSB. Such process is similar to the phase transition (see Figure 02,a) between the gas-liquid-solid phases, in which energy is released to the environment and the rotational symmetry changes to translational with the change of gas/liquid phase to solid. By such analogy, the SSB of electro-weak at 10-12 sec after the Big Bang can be considered as the transition of restmass-less particles moving at the speed of light to motion constrained by having restmass (see Figure 03 of the Standard Model in symmetric and Higgs phases).
Figure 02 Phase Transition	Figure 03 Standard Model	Figure 02,b portrays another interpretation of phase transition via lowering the potential energy V by some kind of catalyzing agent.

Inflaton and Higgs as Metastable States

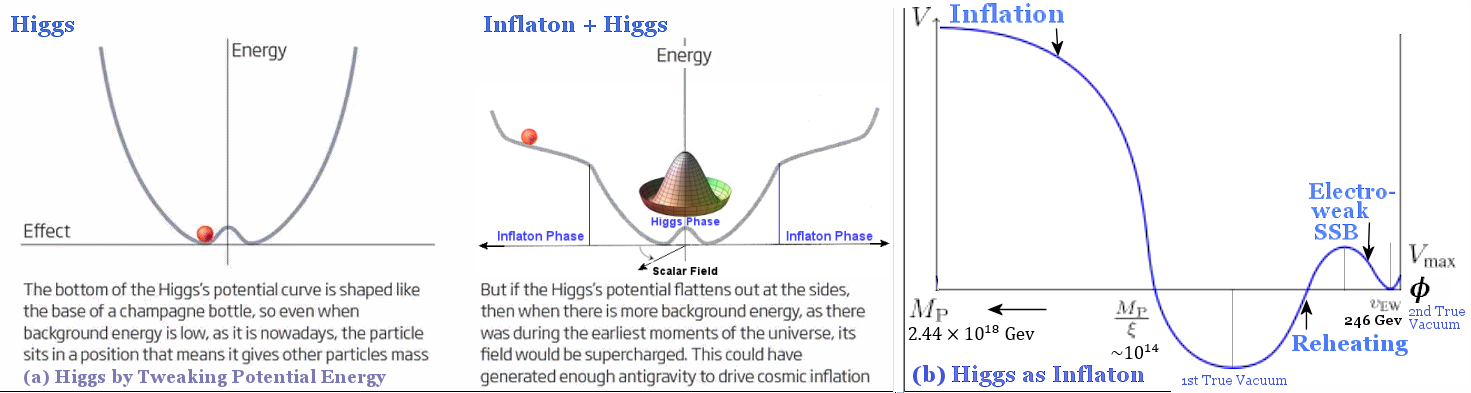The obvious omission in this model is the absence of the reheating process which is responsible for the generation of matter at the end of inflation (see "Inflation Era"). According to another model developed in 2014 (see "Inflation, LHC and the Higgs boson"), it is indeed possible to produce a potential V with an inflationary plateau at large values of the scalar field, and two minima (Figure 04,b).

Figure 04 Higgs as Inflaton [view large image]

A much simplified version of the mathematic derivation is sketched below to illustrate the theoretical formulation.

Figure 05 portrays the time evolution of the inflaton/Higgs field with the potential given by Eq.(3b). It is computed by a constant value of the self-coupling constant . It is also possible to get some idea about each stage of the evolution by inspecting dv/d in Figure 04,b : For dv/d > 0 at large value of , it is in flation era. For dv/d = 0 at the 1st minimum, has reached a true vacuum (Figure 05). For dv/d < 0, it involves reheating and ends up in another false vacuum state. For dv/d > 0 after the 2nd false vacuum, the is making the transition to the final Higgs phase. This is the electro-weak SSB. For dv/d = 0 at the 2nd minimum, this is the SM domain as of today.

Figure 05 Higgs/Inflaton, Evolution with Time

A Semi-classical Model of Inflation (2022 Addition)

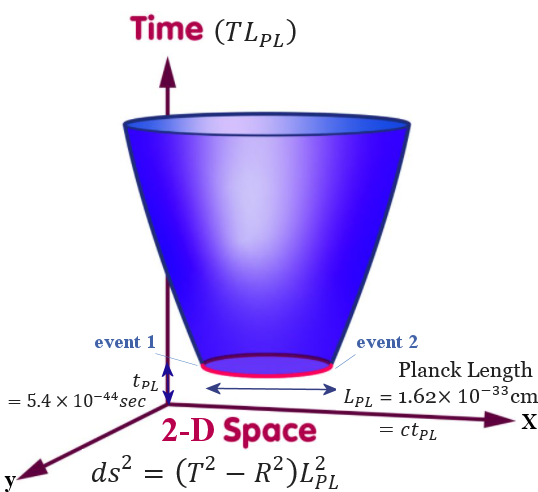
Since inflation occurred at the beginning of the Universe, it is expected that all branches of physics would be combined at this point in a domain called Quantum Gravity (Figure 05a). It would include the gravitational constant G associated with gravity, the Planck constant for quantum, and the velocity of light c in special relativity. All these are encompassed in the system of Planck scale with Planck length LPL = (G/c3)1/2 = 1.62x10-33 cm, Planck time tPL = (G/c5)1/2 = 5.39x10-44 sec, Planck mass MPL = (c/G)1/2 = 2.17x10-5 gm, and Planck energy EPL = (c5/G)1/2 = 1.22x1019 Gev (see "Realm of Planck Scale"). That's why the initial system is postulated to be in such scale at the beginning the Universe.

Figure 05a Quantum Gravity

In addition, LPL and tPL are the absolute minimum space, time. Because any scale below such limit violates the uncertainty principle. For example, EPLt , since EPLtPL = , hence t tPL; similarly for x LPL. (see "What Is The Smallest Possible Distance In The Universe?").

Figure 05b Semi-classical Model [view large image]	According to the consensus view on cosmic inflation, the duration and expanse during that period are : T ~ c(10-32/10-44) ~ 1022, R ~ (10-6/10-33) ~ 1027 and T = R =1, ds2 = 0 time-like in the beginning. Thus, R >> T and ds2 < 0 thereafter, such events are qualified as "Inflation" - QED.

There are many attempts to add quantum into gravity over the course of many decades usually under the umbrella of "Theories Of Everything (TOE)" (see Figure 05c). The "semi-classical, and further ... here", adds one more version into the many alternates. It suggests a rule for quantization (see "Novel Higgs Field"), has no problems with the cosmological constraints, offers an explanation for the dark energy, and is in agreement with the latest measurement of CMB polarization.

Figure 05c Theories Of Everythibg (TOE) [view large image]

Anyway, there is yet another way to achieve the merger by adding gravity to quantum (see "Newtonian Quantum Gravity, 1995", "Newtonian Quantum Gravity, 2006" and Figure 05a).

A Novel Higgs Field

There is an alternate way to associate the Higgs quantum field with cosmic inflation. The formulation starts from a very small universe at Planck scale with Mass/Energy M = MPL and size LPL. Accordingly, its energy density PL ~ 10114 erg/cm3. Such entity is at the realm of quantum gravity. Unfortunately, there is no universally acceptable theory for performing the qunatization of gravity now. One of the ad hoc solutions is to quantize the Friedmann Equation for cosmic expansion. Mathematically, the procedure transforms a non-linear equation such as Eq(5) into a linear equation such as Eq.(6). That is, the procedure displays a continuous scaffolding sheet as divisible units by examining the thing closely (see Figure 05d).

Figure 05d Scaffolding Sheet as QG

Figure 06 H Atom Energy Level & Cosmic Radius	Now the hydrogenic wave function n(R) can be identified to the inflaton field (or the Higgs field , or whatever designation). Inflation corresponds to the transition from the level of n 1 to the continuum (n ). Energy should be infused to pump the transition as shown in Figure 07. After inflation, the

dynamic equation reverts back to Eq.(5) with k 0. The gravity couples only to mass/energy, and detached from the Higgs field.

		Effort to formulate theory in time or size smaller than the Planck scale (~10-44 sec, Figure 08) is doomed to fail as any system below such limit violates the uncertainty principle of t tPL (for the corresponding Planck mass/energy, i.e., EPLt = EPLtPL) and x LPL rendering it meaningless (see "What Is The Smallest Possible Distance In The Universe?").
Figure 07 Cosmic Energy Density [view large image]	Figure 08 Cosmic Evolution	BTW, the cosmic evolution in Figure 08 shows that the structures in quantum domain had completed within a very short time interval about 10-32 sec. Then it went through a period of making the atoms for building the large structures,

A Novel Idea on Dark Energy (2022 Addition)

Figure 08b Dark Energy [view large image]

Meanwhile the quantum fields are also homogeneous, isotropic, and constant over the eon; it is tempting to identify each of the 24 quantum fields (Figure 08b2) to the eigen-function with different value of H (labeled by n, Hn and n for n = 1, 2, 3, ... 24). It is estimated that they would have energy density 10-8 erg/cm3, with the corresponding vacuum (zero point) energy En = hn/2, where n = (k/m)1/2, m is the mass, k the spring constant for the nth particle. The elementary particles in this world are just in the excited state of the quantum fields. As the Universe expands, the quantum fields have to be replenished similarly to the dark energy.

Figure 08b2 Quantum Fields

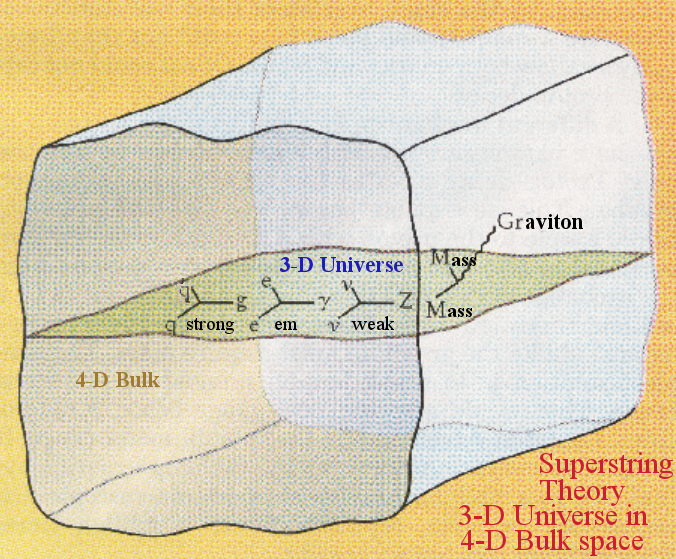As for where does the infusing energy come from ? The quick answer would be from the 4th spatial dimension (Figure 08b3). The corollary for contraction to the beginning is to remove such energy back to the bulk. Thus, such reversal process would not end up with infinite matter-energy as oft-quoted in the past. Unfortunately, the "Superstring Theory" is not viable anymore due to failure of finding any experimental evidence. Or, is the bulk just "Another 3-D World" beyond this Universe ?

Figure 08b3

This is part of the progress in science - more problems are created following the resolution of an old one.

Theories of Cosmic Inflation and B-mode Polarization (2022 Addition)

The pattern on Cosmic Microwave Background (CMB) has shown conclusively that it is the relic of density fluctuation. The theory of cosmic inflation is rather convincingly (not 100%) ascribed as the origin. Mathematically, the CMB (the earliest observable) is expressed by the Power Spectrum : Ps(k) = Ask(ns-1) ---------- (9), where k is the Fourier transform of the spatial coordinate x, ns 1 the scalar index, As the scalar amplitude, the subscript "s" denotes scalar type perturbation (quantum fluctuation), Ps(k) represents the density fluctuation in k-space corresponding to random fluctuation in x-space (see Figure 08c).

Figure 08c Primordial Fluctuations

Some versions of the "Inflation Theory" also include the fluctuation in gravity (or metric tensor in General Relativity paradigm). It is often referred as primordial gravitational wave which is expressed as Pt(k) = Atknt ---------- (10),

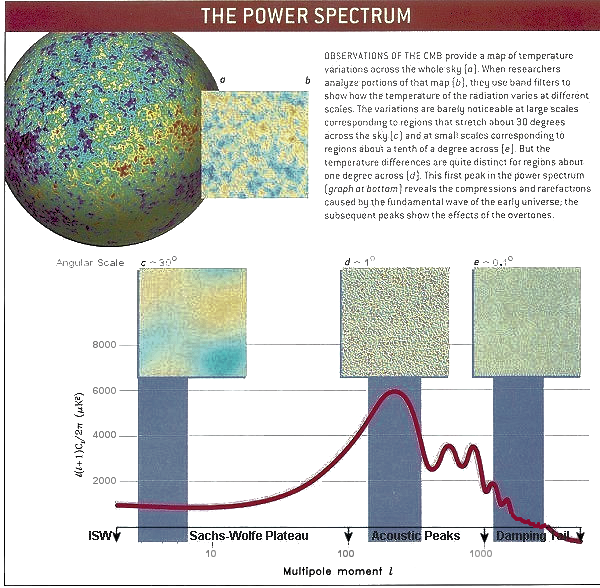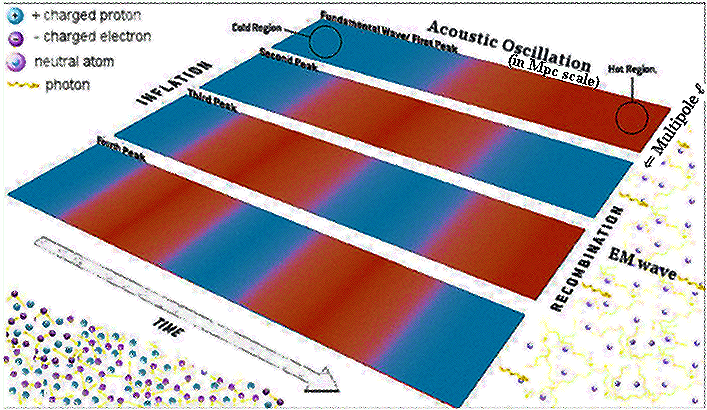
		The gravitational attraction in the density enhanced regions, and the radiation repulsion acted together to produce the incoherent acoustic oscillations (noise). Compressing a gas heats it up; letting it expand cools it down - this is the origin of the temperature variation which eventually shows up as CMB. As shown in Figures 08d and 08e, the main peak is the oscillatory mode that went through 1/4 of a period (reaching maximal compression) at the time of recombination (between electrons and protons to form neutral atoms). The lower peaks correspond to the harmonic series of the main peak frequency.
Figure 08d acoustic oscillations [view large image]	Figure 08e Power Spectrum

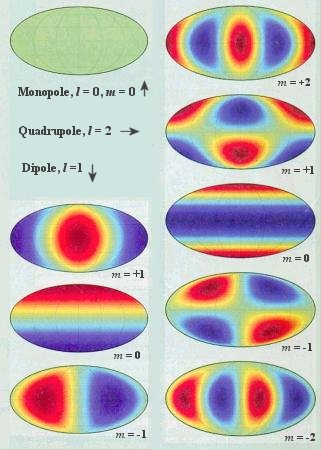In angular coordinates, the temperature fluctuation is expressed mathematically by : where Ym(,), is the Spherical Harmonics, and = 0 denotes the monopole, = 1 the dipole, = 2 the quadrupole, ..., and m can be any integer between - and . Each alm constitutes a multipole mode. The lowest multipoles are the largest-area, continent- and ocean-size undulations on the temperature map. Higher multipoles are like successively smaller-area plateaus, mountains and hills (trenches and valleys) inserted on top of the larger features. The entire complicated topography is the sum of the individual multipoles (Figure 08f).

Figure 08f Multipole

Eq.(11) is the Inverse Fourier Transform back to the x-space with integration replaced by summation over the multipole moment , Ylm playing the role of eikx, and alm the power spectrum Ps(k) in Eq.(9).

	There are two distinct gravitational fluctuations corresponding to two kinds of polarizations as shown in Figure 08g. The E-mode is similar to the scalar fluctuation with g for = = 1,2,3 producing compression/expansion perturbation. While the B-mode is for producing shear perturbation.
Figure 08g Tensor Polarization [view large image]	Thus, the scalar fluctuation generates only E-mode polarization; while the tensor fluctuation (gravitational wave) generates both E-mode and B-mode polarizations.

	Polarization in CMB can be detected by using a polarizer (by the antenna in astronomical instrument). The corresponding formulas to Eq.(11) for CMB in E-mode and B-mode are : where E(n) and B(n) are CMB for the E-mode, and B-mode respectively, and n denotes the direction on the sky (see "Probing Inflation with CMB Polarization").
Figure 08h CMB Spectra, and Polarization Patterns	Figure 08h,a shows the un-polarized, E-mode and B-mode CMB from both scalar and tensor perturbations (dotted curves). The B-mode by lensing is detected from only a small patch of the sky via gravitational lensing. Figure 08h,b shows the patterns around hot and cold spots.

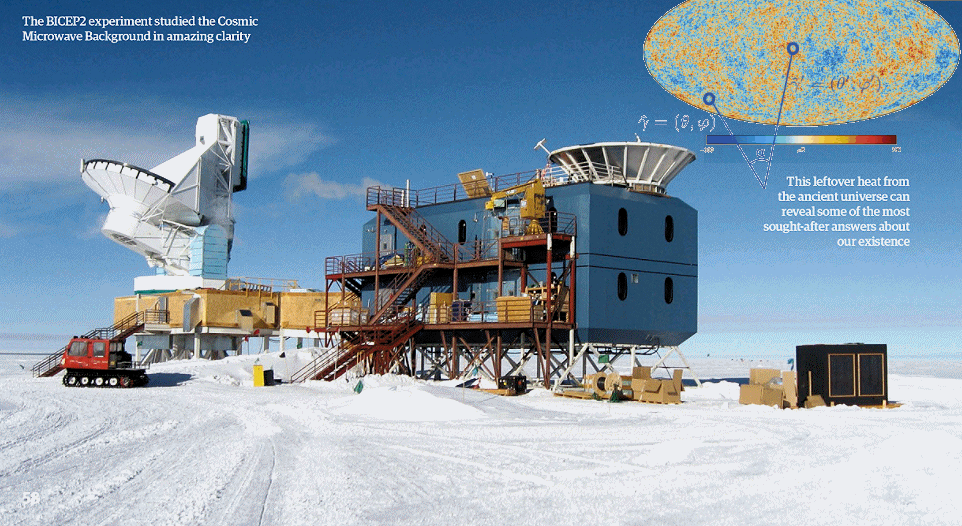
		BICEP2's (Figure 08i) first attempt was a failure because it has not taken into account the inter-galactic dust (see "B-mode Detection, 2010's"). Since then it has created a precise inter-galactic model to subtract out the masking. The 2021 BICEP2 measurement provides the tightest constraint to gravitational perurbation with r < 0.036. The BICEP2 team have arrived at this limit by combining data at three frequencies (96 GHz, 150 GHz, 220 GHz) from their own experiment, complemented by archival data from the WMAP and Planck.
Figure 08i BICEP2 Experiment [view large image]	Figure 08j r-Ratio [view large image]	Figure 08j plots the r-ratio r = Pt/Ps vs the scalar index ns. It encompasses the prediction of some Inflation Models, and experimental measurement (current and future). It is related to the e-fold expansion of space during inflation and is

As for the novel theory of inflation in "A Novel Higgs Field" (see also "Beginning of Time" by the same model in 2019) :

• It is proposed that the wave-function is identified to the Higgs quantum field, which would fluctuated according to the uncertainty principle (see Figure 08k). The fluctuation would in turn produce variation in energy, which can be equated to in the conventional theory of of inflation. Thus, the formulation of the CMB via the variation of would be the same as the case of the variation of the "inflaton" field in the conventional theory; particularly, n_s 1.

Since the commutative rule in the quantization is [-i(d/dR), R] = -i, the scale factor R is also subjected to quantum fluctuation. However it is the diagonal spatial metric, which would generate only E-mode polarization, therefore the tensor to scalar ratio r = 0 for this version of inflationary theory. In addition, the initial size of the inflation is about 10-33 cm (~ Planck length). The inflation terminated when the quantization is no longer valid at about 10-6 cm. Thus the e-fold number N = n[10(-6)/10(-33)] ~ 62 -- close to the valid range of 40 - 60.

Figure 08k Quantum Field

	With such size at the end of the inflation, the total mass M = (10114/c2)(10-6)3 ~ 1075 gm, where the 10114 erg/cm3 is the constant energy density infusion during the period of inflation. This value of M is much greater than the mass of the observable universe ~ 1056 gm implying that the rest of the mass is in the un-observable part of the universe (see Figure 08l).
Figure 08l Cosmic Expansion	The boundary of the un-observable universe for the current epoch ruob can be estimated by : M ~ 1075 ~ 10-29(ruob)3, where 10-29 gm/cm3 is the current matter density, giving ruob ~ 1035 cm (see Figure 08l).

The Schwarzschild radius for M ~ 1075 gm is rs = 2GM/c2 ~ 1047 cm >> ruob indicating that our Universe could be a black hole if the density is concentrated into a much smaller region forming a compact mass. It doesn't seem to be the case by astronomical observations. Anyway, there could be "Another World" beyond ours rendering many cosmic events unfathomable (Figure 08l & 道). Such possibility suggests that human beings are extremely unimportant in the grand scheme of the cosmos as portrayed in a 1985 movie called "Insignificance" in which Einstein and Monroe explore relativity and our place in the Universe (Figure 08m).

Figure 08m Insignificance [view large image]

• Here's the resolutions for the three problems in Big Bang cosmology by the novel model (Figure 08n) :
  
  
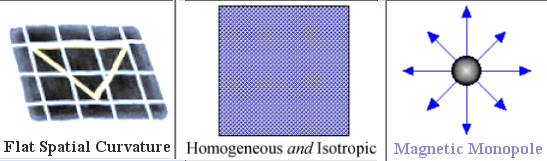
  1. Flat Spatial Curvature - The spatial curvature is too small to be measured by both the WMAP and Planck satellites. It is estimated by comparing the total density parameter with the critical one that leads to the conclusion of near nearly flat spatial curvature for this universe. However, WMAP has assessed the radiation density parameter _rad = 8.24x10^-5 implying that the curvature density parameter _k < 10^-5. Since _k = kc²/(H₀)², for the Hubble constant H₀ = 72 (km/sec)/Mpc,
     k < 10^-62 cm^-2. According to Eq.(7) the initial spatial curvature (with n = 1) is k₀ 10⁶⁶ cm^-2, it has diminished ~ 10¹²⁸ fold during the period of inflation.

  	Homogenous and Isotopic Space - Since the novel model starts with Planck size, by definition of Planck scale there would have just enough time for signal traveling at the speed of light to cover distance of the length of the diameter. The whole system would be thermalized at the beginning and carried over ever since.
  Figure 08n The 3 Problems [view large image]

  2. Missing Magnetic Monopoles - This is a problem with Grand Unified Theories (GUT), which propose that at high temperatures (such as in the early universe) the electromagnetic force, strong, and weak nuclear forces are not actually fundamental forces but arise due to spontaneous symmetry breaking from a single Gauge Theory. These theories predict a number of heavy, stable particles that have not been observed in nature. The most notorious is the magnetic monopole, a kind of stable, heavy "charge" of magnetic field. Monopoles are predicted to be copiously produced following Grand Unified Theories at high temperature, and they should have persisted to the present day, to such an extent that they would become the primary constituent of the Universe.
     The novel model has superseded the GUT, and hence no magnetic monopoles are produced (see Figure 08) .
  
  

  Furthermore, Entropy is defined as the degree of randomness, which can be expressed alternatively as the degree of freedom in a system, i.e., the number of different parameters or arrangements needed to specify completely the state of a system. The evolution of entropy in the universe can be separated into four phases as described in Figure 08o, which shows lot of (???) for the very low entropy at the beginning of the universe. It has been a puzzling problem since a hot Big Bang should involve a lot of entropy (randomness/disorder). The mathematics in the above quantum cosmological model is specified by only "one" parameter, i.e., the Planck length LPL, which is the absolute minimum entropy for any system. Thus, low entropy came naturally in the model resolving the paradox.

  Figure 08o Evolution of Entropy [view large image]

(Modified) Quantum Field History

The universe starts with gravity (which goes on by itself) and a quantum wave function that is identified as the Higgs field. This Higgs field went on to undergo many phase changes and finally settled down with 3 distinct quantum fields plus the Higgs field itself as shown in Figure 09. Such phenomena is similar to the phase transition of water (see Figure 02), which can exist in the form of gas, liquid, and solid in macroscopic states (similar to the strong, weak, and em quantum fields; or like mother and children).

Figure 09 Quantum Field History (modified) [view large image]

NB : The gravitational field is a classical field that does not follow the rules for the quantum fields. It only coupled to the Higgs field at the very early cosmic time in the form of quantum gravity. It detaches from the Higgs after inflation when the size of the universe is beyond the quantum domain.

The role of the Higgs field in the different phases is discussed briefly in the followings :


1. Planck Scale (the Constants) - Soon after Max Planck introduced the Planck constant h in 1899 to account for the spectrum of blackbody radiation, he realized that the only way to construct a unit of length out of h = 6.625x10^-27 erg-sec, the velocity of light c = 3x10¹⁰ cm/sec, and the gravitational constant G = 6.67x10^-8 cm³/sec²-gm, is L_PL = (Gh/c³)^1/2. This is the Planck length.

   Subsequently, it transpires that the realm of Planck scale would involve entities with size of the order 10-33 cm and associated with high energy phenomena at 1019 Gev (see list below). Such environment is considered to occur at or near the moment of Big Bang - a most favorable theory for the creation of this universe. Here's the Planck scale :

   Planck Length - LPL = GMPL/c2 = (G/c3)1/2 = 1.62x10-33 cm. Planck Time - tPL = LPL/c = (G/c5)1/2 = 5.39x10-44 sec. Planck Mass - MPL = (c/G)1/2 = 2.17x10-5 gm. Planck Energy - EPL = MPLc2 = (c5/G)1/2 = 1.22x1019 Gev = 1.95x1016 erg. Planck Temperature - TPL = EPL/kB = (c5/GkB2)1/2 = 1.42x1032 oK. Planck Energy Density - PL = (c7/G2) ~ 10114 ergs/cm3. Note that at speed of light c, there is enough time tPL for signal to travel the length of LPL, i.e., no problem with homogeneous/isotropic of the universe; and EPLtPL = satisfies the Uncertainty Principle.

   Figure 09b Convergence of Quantum Gravity [view large image]

   Figure 09b shows all kinds of objects in this world with different size and mass. Ultimately, they all emerge from a tiny speck at the Planck scale via the action of Quantum Gravity (see the corresponding Table).

   
   
2. Planck Era (the Theory) - Theoretically, the moment of Big Bang should be related to General Relativity, specifically, the Friedmann Equation in Standard Cosmology. This equation has to be quantized if the initial entity is in Planck scale as discussed in "Novel Higgs Field". It turns out that the wave function is similar to the Hydrogen atom's with the scale factor R as independent variable. This wave function is identified to the Higgs field . The transition of from the state of huge curvature k to k = 0 represents the period of inflation at the end of which it approaches an oscillatory form ~ Ae^-i(t-kx), which is the solution for the "Klein-Gordon Equation of the Scalar Field". At this stage, the Higgs field could be the only quantum field around. It used to be called "Grand Unification" by assuming that all the quantum fields are in existence all the time but merged together at this point.
   BTW, the "flatness" problem is resolved by the transition of curvature k ~ 1/L_PL to k 0 in the quantum cosmological model.



Reheating and "Electroweak Unification" - During inflation, the environment lost a lot of energy in the process. Reheating is another process whereby the energy of the environment is replenished by energy from the Higgs field in the form of quarks and gluons (the Quark-Gluon Plasma QGP, see Figure 10). Separation of the quark fields (of strong interaction) at this period represents a form of phase transition. The re-shuffling also moves the Higgs field to a false vacuum state (mathematically by adding the potential V() to the Klein-Gordon Equation, see Eq.(3b)).

Figure 10 Quark-Gluon Plasma [view large image]

N.B. Reheating is not necessary, if the energy infusion is not from the immediate environment (see "Another World").

3. Electroweak Spontaneous Symmetry Breaking (SSB) - At 10^-12 sec after BB (Figure 09), the Higgs field decays from the false vacuum to the true vacuum precipitating another phase transition. According to the Standard Model (SM, Figure 11), all the quantum fields already were in existence at the state of false vacuum. However, the quantum fields were not interacting with the

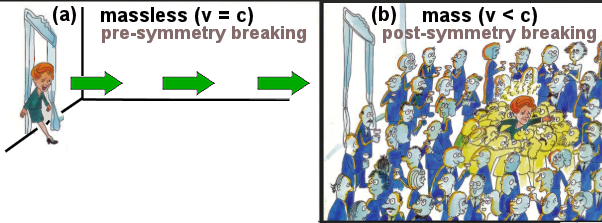   		Higgs and hence the quantum particles (excited from the fields) are restmass-less moving at the velocity of light (Figure 12). Most of them acquired restmass only after SSB. The event separated the electroweak field into the electro-magnetic and weak; it also made the left/right-handed versions of the quarks and leptons acting differently in weak interaction.
   Figure 11 SM, Parameters	Figure 12 Mass, Origin of [view large image]	Another phase change occurs about 10-5 sec after BB. This one transforms the Quark-Gluon Plasma to the bound state of Hadons (see "Chiral Symmetry Breaking").

   BTW, BB here denotes "Big Bang" not "Best Before" (no pun intended).



	By taking into account the higher term of the SM (Standard Model) perturbation series, the vacuum potential of the Higgs field V is in the form :
Figure 13 Stability of the Universe [view large image]	and the sum is over all SM particles acquiring a Higgs-dependent mass Mi. The precise form of V1 is not important in the present context, it just shows that the Higgs potential also depends on the particles it acts upon. Furthermore, only the heaviest top quark in the sum is retained in the following consideration.

As shown in Figure 13,a and the above formula, the vacuum potential V depends on the mass of the Higgs m and also the mass of the top quark m_t. Computation of V with the various values of m and m_t yields different forms, which can be stable, meta-stable, or runaway (see Figure 13,a). Figure 13,b plots the result revealing our universe is possibly in an meta-stable state sitting on the verge of catastrophic decay into the true vacuum. The revelation produced sensational news headline around the globe one morning in 2013 (soon after the discovery of Higgs in July, 2012). A second wave of doomsday prophecy occurred in 2014 with Hawking's blessing on the revelation.

Quantum Field History à la Gauge Theory

The concept of gauge invariance (or gauge symmetry) can best be visualized with an example, which portrays a boat floating on the water surface with the depth of water at 3 meters (Figure 14). The gauge (the water level marker) at the left registers a reading of 6 meter on the surface and 3 meter on the bottom, while the measurements from the other one at the right are 9 and 6 meter correspondingly. The differences in the readings are the result of moving the right marker further down, but the depth of water remains the same at 3 meters - the picture has not been changed by moving the gauge around. In theorietical physics it is the governing equation that is unchanged (invariant) under the change of some parameters, which turn out to be the phase angles in an abstract space. When the system changes during the process of measurement, e.g., the boat sinks to the bottom during a storm, the symmetry is borken, i.e., it is not the same before the measurement. If it occurs naturally, then it is said to be Spontaneous Symmetry Breaking (SSB).

Figure 14 [view large image] Gauge Invariance

In Gauge theory, the Largangian or field equation is invariant under the gauge transformation : ' = ei(at) , where a is the phase angle associates with the "axis", t the generator in the form of nxn matrix, n the dimension of the Gauge space, the number of phase angles is determined by the formula Na = n2 - 1 (except for n = 1, Na = 1, see a little bit more in "Unitary Groups"). Figure 15 is an example of simplest Gauge transformation with n = 1. It shows that the SO(2) rotational transformation of 2-component field (interpreted as two +/- charge states) is equivalent to a single complex field in U(1)= eiI (I = 1) Gauge group. In general SO(n+1) ~ SU(n), where "S" means that the transformation is "Special" with |det(U)| = +1, which implies continuous rotations without reflection.

Figure 15 [view large image] Gauge Transformation

This is "Global Gauge Transformation" applicable to all space. It is the spatial dependence "Local Gauge Transformation" that generate the Gauge bosons responsible for interaction of particles.

The followings trace the variations of the Gauge environment at different phase of the Quantum Field Evolution :


1. Planck Scale Era (novel) :
   In the "Novel Quantum Cosmologic Model", the cosmic wave equation Eq.(6) is global Gauge invariant with ' = eⁱ , where is a constant, while the wave function is identified to the Higgs field up till the end of inflation.


2. Grand Unified Theory (conventional) :
   • As shown in Figure 15b, all quantum fields are in absolute zero ground state. There are virtual particles filling up the space in quantum fluctuation incessantly according to the uncertainty principle, but real particle only appears with infusion of suitable amount of energy. However, it would be restmass-less and flying around at the speed of light. It is not know exactly how, and

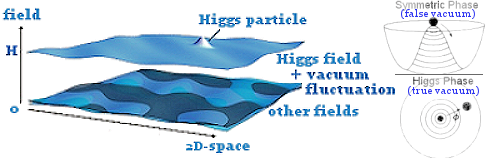     	why, and when, but in certain circumstance (probably via decreasing temperature caused by cosmic expansion), the Higgs field would develop to a false vacuum state and then decays to the state of true vacuum H (as depicted in Figure 15b). Then the particles would interact with the Higgs field to slow down by exchange of energy and appear as acquiring mass.
     Figure 15b Higgs Field [view large image]	It is not known whether the quantum fields are inherent to the initial environment or developed gradually. Regardless of either possibility, one Gauge theory assumes that it started with a more encompassed Gauge group namely the SU(5) (also see "Group").

   • The SU(5) is the simplest Gauge group which includes all the quantum fields and bosons in the Standard Model (SM) plus some more.
   • Similar to the symmetry group of sphere that contains circles as its subgroups, the SU(5) group contains the subgroup
     SU(3) x SU(2) x U(1).
   • The elementary particles are assigned into two 5-multiplets and two 10-multiplets (Figure 16,a). Figure 16,b shows one of the 5-component multiplets with the 24 gauge bosons assignment arranged into a matrix. Of these, 12 are familiar (the W⁺, W^-, B and 8 gluons). The remaining 12 are new bosons denoted by X and Y, The mass of the X bosons have been calculated under the Higgs mechanism, and turns out to be about 10¹⁵ Gev. These super-heavy particles lie many orders of magnitude beyond the energy ranges of any conceivable accelerator. However, they could be present in great abundance in the GUT Era

     	about 10-35 sec after the Big Bang. These additional bosons carry new forces which can transform quarks into leptons and vice versa (hence the proton decay, which failed to be confirmed experimentally leading to the desertion of the theory).
     Figure 16 GUT, SU(5) [view large image]	Moreover, the theory also predicts the existence of magnetic monopole, which is also not observed in nature. See the more encampassing SO(10) Group.

   • It requires 2 Higgs fields, the H⁺ and H⁰ (in an additional 5-component multiplet) to break the unification to SU(3) x U(1)_em when the Higgs decays from false to true vacuum (See "Georgi-Glashow model"). This is the transition to break the strong interaction from the electroweak field about 10^-34 sec after BB (see Figure 09). Such process repeats itself later in breaking up the Electroweak Unification and also in the Chiral Symmetry Breaking. This kind of process is very similar to the transition from one meta-stable state to another meta-stable state in lower energy. However, the mechanism for transition is neither quantum tunneling nor through some catalyzing agents; it is instead via the development of the unstable false vacuum (see Figure 17 for the essential steps).
   
   
3. Spontaneous + Electroweak, Symmetry Breaking (SSB + ESB) :
   • As the electroweak-strong interaction splitting settled down to the true vacuum state. It can be considered as another era of stable environment where the Higgs has Energy density E = M²² + ⁴ (aka potential energy V), where the mass M > 0 and the self-coupling constant > 0 (see Figure 17, upper right).
   • The existence of the electroweak interaction has been verified experimentally by neutrino scattering and subsequent discovery of the W and Z Gauge bosons (see "Electroweak interaction").
   • In the symmetric phase, the electroweak composes of both left and right handed leptons and quarks in SU(2) symmetry plus 4 gauge bosons (Figure 17, upper left and middle).
   • Then some mechanism (probably caused by the lowering temperature) at 10^-12 sec after BB transforms either the mass M² to become negative (i.e., it becomes Tachyon), or the self-coupling becomes replusive (i.e., < 0). The Higgs becomes unstable after such transformation; it is said to be in "false vacuum".
   • Spontaneous Symmetry Breaking (SSB) is the process of Higgs field transition from the false to true vacuum which is in a stable state of lower energy.
   • The process of SSB turns (W¹, W²) to (W⁺, W^-); and (W³, B) to (Z, ). This is the link between SSB and ESB since such

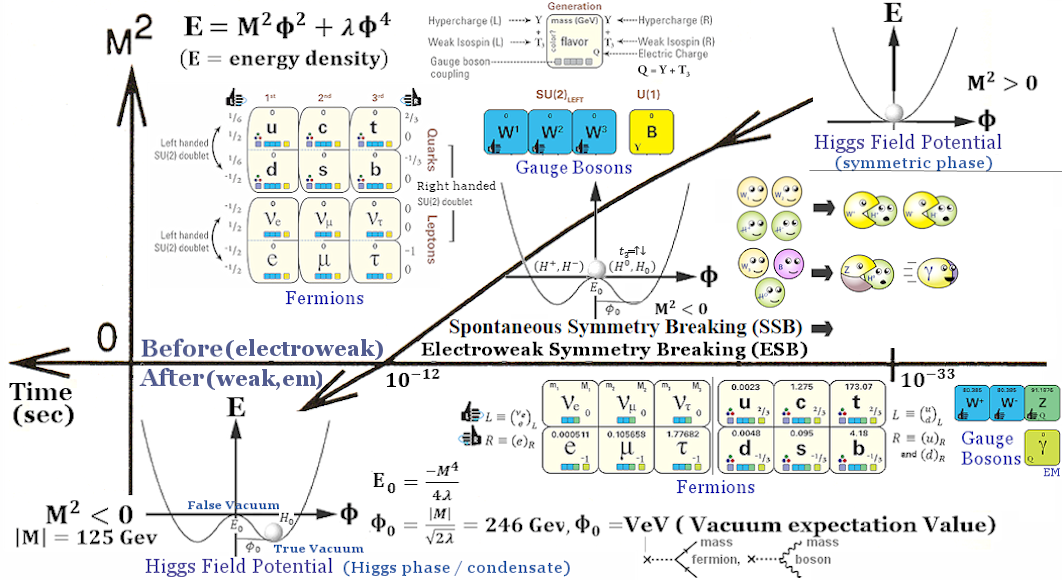     transformation of electroweak bosons to (W+, W-, Z) bosons involves the combination (eating as shown in Figue 17) with the three Goldstone bosons from the Higgs to acquire the longitudinal polarization (equivalent to endowing mass). The transformation also breaks the left/right handed symmetry. The left handed one still has SU(2) gauge symmetry running both weak and electromagnetic interactions; while the right handed version is relegated to have U(1) symmetry, (a singlet without neutrino) interacting via electromagnetic only.

     Figure 17 SSB + ESB [view large image]

     Without further ado, here's the mathematics to show the consequence of SSB using a simplified example of one boson field (see "Spontaneous Symmetry Breaking" for further details of the mathematics) :

     
     
     
   • The matter/anti-matter asymmetry was also produced around this time frame (see "leptogenesis").
   
   
   • Update from "New type of dark energy could solve Universe expansion mystery" :
     In September 2021, two independent reports from Atacama Cosmology Telescope (ACT) and South Pole Telescope (SPT) indicating that their data are consistent with the theory of "Early Dark Energy (EDE)" in addition to the standard dark energy. The EDE would not be strong enough to cause an accelerated expansion, but it would have caused the plasma that emerged from the Big Bang to cool down faster than it would have otherwise. Interpreting the CMB data on the basis of EDE and ACT data would reduce the age of the Universe to 12.4 Gys, about 11% younger than the 13.8 Gys calculated using the standard model. While the Hubble constant is H₀ ~ 71 (km/s)/Mpc in favor with the local measurements. The EDE model is also consistent with the SSB/ESB mentioned above. There is a constant supply of energy during the Higgs field transition from the false to true vacuum state. The cooling and reheating is the same as the inflation, albeit in a much smaller scale.
   
   
4. Spontaneous + Chiral, Symmetry Breaking (SSB + CSB) :
   • This is yet another false to true vacuum SSB about 10^-5 sec after BB. Then the Chiral Symmetry Breaking transforms the quark-gluon plasma to bound systems of hadron. At the end of the process, it generates 95% of the hadron mass (from the energy that binds the quarks together, Figure 18b,b). The process also produces the pions, the virtual particle of which provide the "residual" nuclear force between nuclei.

   The Lagrangian for QCD LQCD in SM is shown on the bottom of Figure 18a. In early universe up to 10-12 sec after BB, the fermions (u,d,s,c,b,t) are massless, i.e., mi = 0. They couple to the SU(3) Gauge bosons Ga, (where the index "a" runs from 1 to 8) as well as the SU(2) Gauge bosons according to the Standard Model (see Figure 11). The system of quarks and gluons flew at the speed of light as some sort of fluid. This state of the QCD can be considered as free relativistic gas called Quark-Gluon Plasma (QGP, see "Quark-Gluon Plasma and the Early Universe").

   Figure 18a SU(3) Gauge Theory [view large image]

   	In the symmetric phase, the quantum fields for strong interaction consist of both left and right handed quarks in SU(3) triplets plus 8 gauge bosons. The quarks and gluons are restmass-less and free to move at speed of light as quark-gluon plasma (see Figure 18b,a).
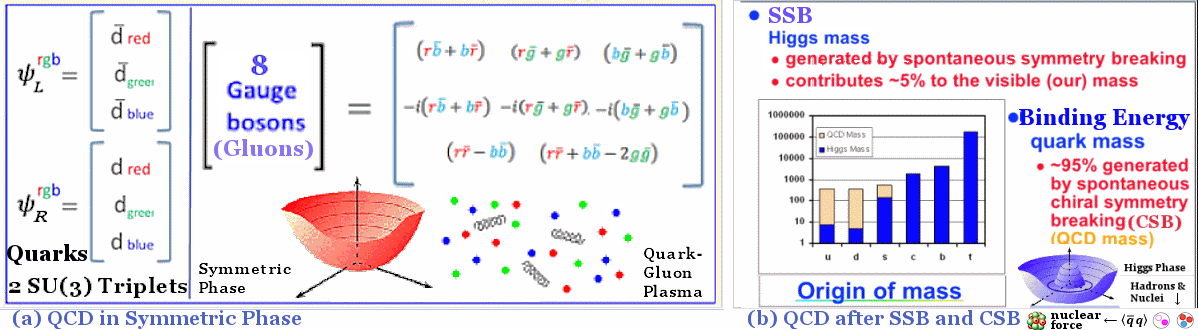   Figure 18b SSB + CSB [view large image]	After SSB and CSB, there is only 1 flavor triplet for each quark, 8 gluons and 3 pions (0, +, -) which are the 3 Goldstone bosons from the Higgs with mass contributed by CSB. The pions have minimal mass among all mesons (see "List of Mesons and Meson").

   • It turns out that the simple evolutionary path (see Figure 10 and the thin green line in Figure 19) for Quark-Gluon Plasma (QGP) and Hadron Gas is applicable only for crossover (smooth) transition at the Hagedorn temperature T_H. As the temperature drops below T_H, the process has to go through a phase transition as shown in Figure 19.
     • There are two ways to describe the transformation : a) high temperature; and b) high baryon density. These two distinct limits are smoothly connected and that the phase boundary is a smooth line in the _B-T plane (for baryon, Figure 19).
     • The chemical potential is a form of potential energy that can be absorbed or released during a chemical reaction or phase transition. It is a function of density, temperature, pressure, ... (see insert in Figure 19 for a simple example). At constant temperature and pressure, the Gibbs free energy G (energy releases to or absorb by the environment) is expressed by :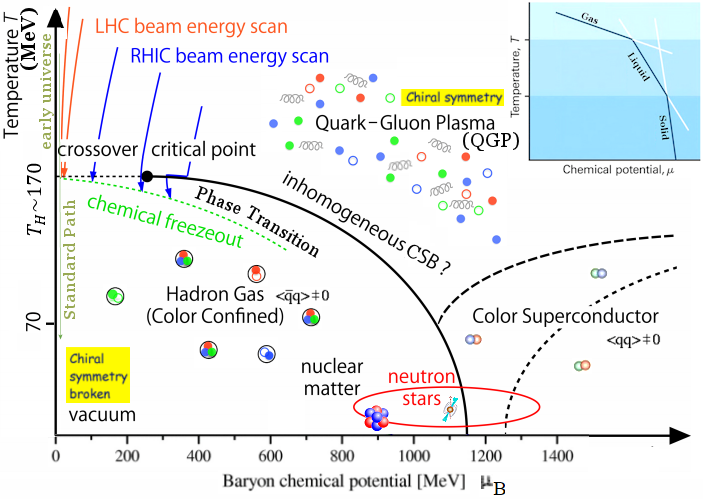
       

       Figure 19 QGP/Hadron Gas Transition	Starting about 50 years ago, experiments using Relativistic Heavy Ion Collisions (RHIC) have demonstrated the existence of QGP (see review article "Melting Hadrons, Boiling Quarks"). All these studies obtain deconfinement through high temperature (Figure 19).

The main way of processing crude oil is to separate it using fractional distillation. Oil is made up of many different hydrocarbons of different boiling points (Quantum Fields). The first step in distillation is to boil the oil to a very high temperature (Big Bang), usually around 400oC. When the solution boils, it forms vapors entering the fractional distillation column (GUT), which has many trays to collect the liquid (Quantum Fields). As the height in the column increases, the temperature gets cooler, so as the vapors rise, they cool. Since every hydrocarbon chain has a different boiling point, as a chain reaches a height where the temperature is lower than the boiling point, it will cool into a liquid and be collected by a tray (Symmetry Breaking Processes). This method allows for all parts of the oil to be separated and collected so that they can be further processed and refined (Standard Model). A very good animation of the distillation process can be found in Figure 20.

Figure 20 Q Fields Evolve and Crude Oil Distillation

According to this scenario, all the quantum fields were unified (mixed together) in the beginning. Each one emerges from the whole and manifests its characteristics only at suitable temperature by the process of Symmetry Breaking. Ultimately, it is the cosmic expansion (which keeps lowering the temperature) that causes all these cosmic phase changes (Figure 09).